Sometimes easy and sometimes hard, our calculus problem of the week could come from any calculus topic. If you really want to get better at calculus, following these problems is a great way to make yourself practice! Past calculus problems of the week.
This week’s problem:
(click “see the solution” at the bottom of post to, well, see the solution.)
Find the integral (as far as you can): 
A first thought may be to distribute the 
, but after that step it is unclear how to proceed! Instead, notice that we have a product of two functions, namely 
and . This is a good indication that integration by parts would be a helpful approach!
To apply this, we have to decide what
and 
are. Clearly if we take the derivative of f(x) again we will be moving in the wrong direction by making things more complicated. Also, it is really easy to take the derivative of 
which we will have to do with whatever we choose as . Therefore, a good choice is:
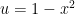
Now, to use the formula we must find
and 
. In this case,
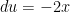(took the derivative of u)
Finally, we get
The second integral looks like a simplified version of the one we started with and just like before, integration by parts will allow us to find this integral. If
and 
then:
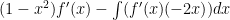
After all of that, this as far as you can go. We don’t know enough about f(x) to know what the last integral is but we were able to take the original integral and simplify it down a bit. As you can see, this involved quite a few steps, so if there is one that doesn’t make sense please feel free to ask!
, but after that step it is unclear how to proceed! Instead, notice that we have a product of two functions, namely and . This is a good indication that integration by parts would be a helpful approach!To apply this, we have to decide what
and are. Clearly if we take the derivative of f(x) again we will be moving in the wrong direction by making things more complicated. Also, it is really easy to take the derivative of which we will have to do with whatever we choose as . Therefore, a good choice is:
Now, to use the formula we must find
and . In this case, (took the derivative of u)
Finally, we get
The second integral looks like a simplified version of the one we started with and just like before, integration by parts will allow us to find this integral. If
and then:
After all of that, this as far as you can go. We don’t know enough about f(x) to know what the last integral is but we were able to take the original integral and simplify it down a bit. As you can see, this involved quite a few steps, so if there is one that doesn’t make sense please feel free to ask!