Calculus Problem of the Week September 16 2011

Sometimes easy and sometimes hard, our calculus problem of the week could come from any calculus topic. If you really want to get better at calculus, following these problems is a great way to make yourself practice! Past calculus problems of the week.

This week’s problem:
(click “see the solution” at the bottom of post to, well, see the solution.)

Find the integral: \int\dfrac{5\sin^3{x}\cos{x}}{1-\cos^2{x}}dx.

See the solution.

What I’m hoping catches your eye is the denominator of 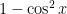1-\cos^2{x} – for two reasons. First of all, with this denominator there, it is unclear how to proceed with the integral. Secondly, you should have the identity

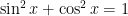
\sin^2{x}+\cos^2{x}=1

BURNED into your brain! Using this identity, you can rewrite the entire integral.

\int\dfrac{5\sin^3{x}\cos{x}}{1-\cos^2{x}}dx=\int\dfrac{5\sin^3{x}\cos{x}}{\sin^2{x}}dx=\int5\sin{x}\cos{x}dx

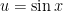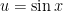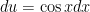Now this integral can be found using the substitution u=\sin{x}. If u=\sin{x}, then du=\cos{x}dx and dx=\dfrac{du}{\cos{x}}. Then the integral becomes:
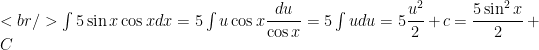
<br /> \int5\sin{x}\cos{x}dx=5\int u\cos{x}\dfrac{du}{\cos{x}}=5\int udu=5\dfrac{u^2}{2}+c=\dfrac{5\sin^2{x}}{2}+C

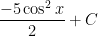Note: You could have used u=cos(x) and your final answer would be \dfrac{-5\cos^2{x}}{2}+C. Now, this does not EQUAL the above answer but they differ only by a constant which is accounted for in C.